Passive directors

How do small non-spherical particles move relative to each other in a turbulent flow? It is plausible that turbulent strains align the particle orientations as the particles approach, and in this case one expects that particle orientation is a smooth function of particle position.

But experiments reveal that the orientation patterns of non-spherical particles in turbulence are not smooth in general, not even at the smallest scales where the turbulent fluid velocities are smooth functions of position.  It turns out that  angles between the orientations of nearby particles are anomalously large (Figure 1).

Using  statistical-model simulations we demonstrated that the attractor determining the particle-orientation patterns is fractal, and that the steady-state distribution of angles between nearby particles has power-law tails. We derived a theory based on diffusion approximations that can at least qualitatively explain these observations. We found that singularities in the particle-orientation field contribute to the anomalously large angles. There are intriguing connections to the statistics of velocities in Burgers turbulence, but we have not yet managed to derive a quantitative theory.

The results are of general importance for multi-phase flows, because they indicate that non-spherical particles advected by turbulence tend to approach at larger relative angles than commonly thought.  This affects the rate at which the particles collide, and possibly also collision outcomes. We also observed singularities in the particle-orientation field that

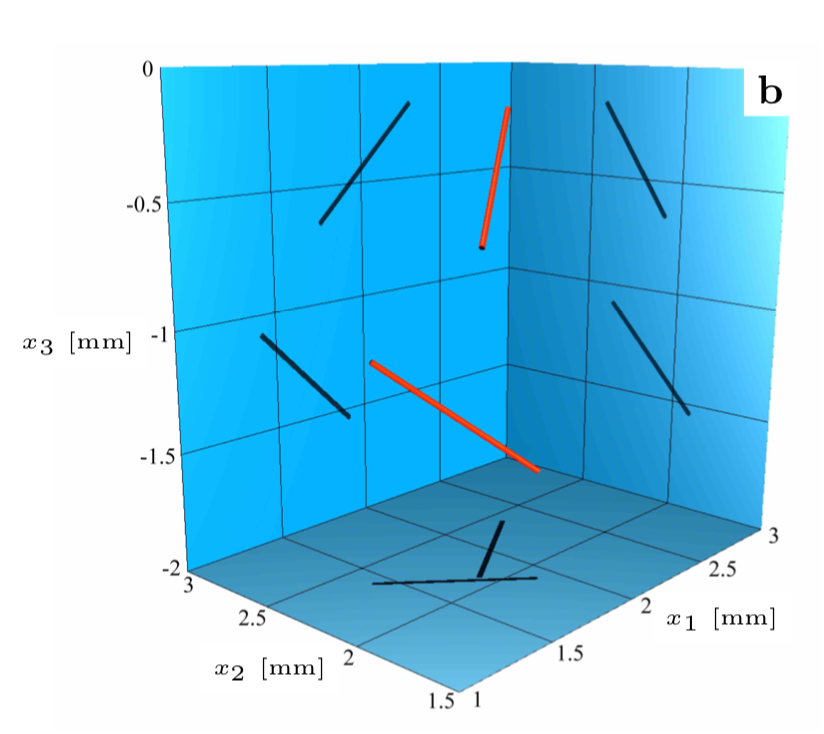
Figure 1. Reconstruction of the orientation of two nearby rod-like particles (red) from experimental data obtained by observing small segments of fishing-line in a turbulent water tank. From Ref. [1].

[1] Passive directors in turbulence
L Zhao, K Gustavsson, R Ni, S Kramel, GA Voth, HI Andersson & B Mehlig, Physical Review Fluids 4 (2019) 054602

[2] Emergent scar lines in chaotic advection of passive directors
B Hejazi, B Mehlig & GA Voth, Physical Review Fluids 2 (2017) 124501

[3] Emergent order in rheoscopic swirls
M Wilkinson, V Bezuglyy & B Mehlig, Journal of Fluid Mechanics 667 (2011) 158-187

[4] Poincaré indices of rheoscopic visualisations
V Bezuglyy, B Mehlig & M Wilkinson, Europhysics Letters 89 (2010) 34003

[5] Fingerprints of random flows?
M Wilkinson, V Bezuglyy & B Mehlig, Physics of Fluids 21 (2009) 043304